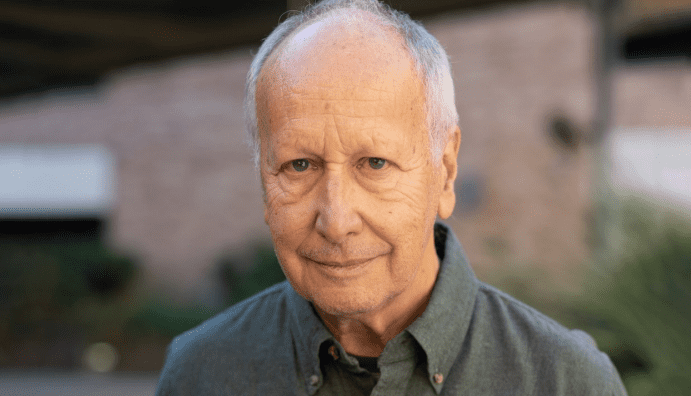
Luis A. Caffarelli, an Argentina-born mathematician, has been awarded the Abel Prize, also known as the “Nobel” of mathematics, for his contribution to regularity theory for nonlinear partial differential equations (PDEs). PDEs are a type of equation that models how specific variables change with respect to each other, making order out of the chaos in the real world. Caffarelli made significant contributions to characterizing singularities and working on free-boundary and obstacle problems, which are important in physics, economics, and other fields. He is one of the most prominent living mathematicians, with over 320 papers, more than 130 collaborators, and over 19,000 citations. He continues to be active in the field, publishing several papers a year and collaborating with graduate students and colleagues.
The Abel Prize Awarded to Luis A. Caffarelli for Contributions to Partial Differential Equations
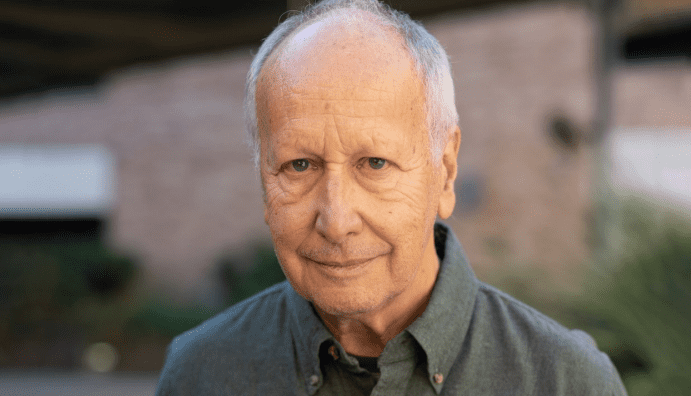
Luis A. Caffarelli, an Argentina-born mathematician, has been awarded the Abel Prize, often referred to as the “Nobel” of mathematics. The prize, named after Norwegian mathematician Niels Henrik Abel, is awarded annually to one or more outstanding mathematicians by the King of Norway.
Caffarelli was celebrated for his “seminal contributions to regularity theory for nonlinear partial differential equations.” Partial differential equations (PDEs) are a type of equation commonly used in modeling physical processes. They model how specific variables change with respect to each other, making order out of the seemingly chaotic nature of the real world.
While mathematics is essential to virtually all fields of science, it doesn’t have a Nobel Prize. Instead, it has the Fields Medal, awarded every four years to leading researchers under 40, and the Abel Prize. The latter is modeled after the Nobel Prize and recognizes the contributions of outstanding mathematicians like Caffarelli.
Differential equations, which are used to measure change, are the basis for much of Caffarelli’s work. These equations relate one or more unknown functions and their derivatives, with the functions generally representing physical quantities and the derivatives representing their rates of change. For example, speed is the first derivative of distance with respect to time, and flow is commonly described with differential equations.
Caffarelli’s work introduced new techniques and produced seminal results that advanced our understanding of how differential equations can be used in multiple areas of mathematics and physics. This is particularly important because straightforward techniques don’t always work, and applications can become complex quickly.
In summary, Caffarelli’s contributions to regularity theory for nonlinear partial differential equations have earned him the prestigious Abel Prize, highlighting the importance of mathematics in understanding the world around us.
Luis A. Caffarelli’s Contributions to Characterizing Singularities, Free-Boundary Problems, and Obstacle Problems
Luis A. Caffarelli, the recipient of the Abel Prize, made significant contributions to various fields of mathematics, including characterizing singularities and working on free-boundary and obstacle problems. Singularities refer to mathematical points at which an object is not well-behaved, which is important in physics and other areas where it’s hard to characterize the behavior of physical systems. Free-boundary problems, on the other hand, occur at boundaries where one thing turns into another, such as the transition from water to ice or from liquid to crystal. They also play a significant role in economics. Caffarelli worked on a specific type of boundary problem called an obstacle problem, which is useful in gas and liquid flows in porous media and financial mathematics.
Caffarelli, who was born and grew up in Buenos Aires and worked mostly at US universities such as the University of Minnesota, the University of Chicago, New York University, and Princeton, published extensively throughout his career, with over 320 papers and more than 130 collaborators. His papers were well-received, with over 19,000 citations, and he had over 30 Ph.D. students, including Fields Medalist Alessio Figalli.
At 74, Caffarelli continues to be active in the field, publishing several papers a year and collaborating with graduate students and colleagues. His work has significantly advanced our understanding of partial differential equations (PDEs), making him one of the most prominent living mathematicians in this field.
Don’t miss interesting posts on Famousbio